Dive into the realm of Algebra 2 12.2 Worksheet Answers, where mathematical concepts dance with real-world applications, forging a path towards academic excellence. This comprehensive guide empowers learners to unravel the intricacies of algebra, unlocking the secrets to problem-solving success.

Through engaging explanations, practical examples, and expert guidance, this narrative unravels the significance of understanding algebraic concepts for academic achievement and beyond.

Algebra 2 12.2 Worksheet

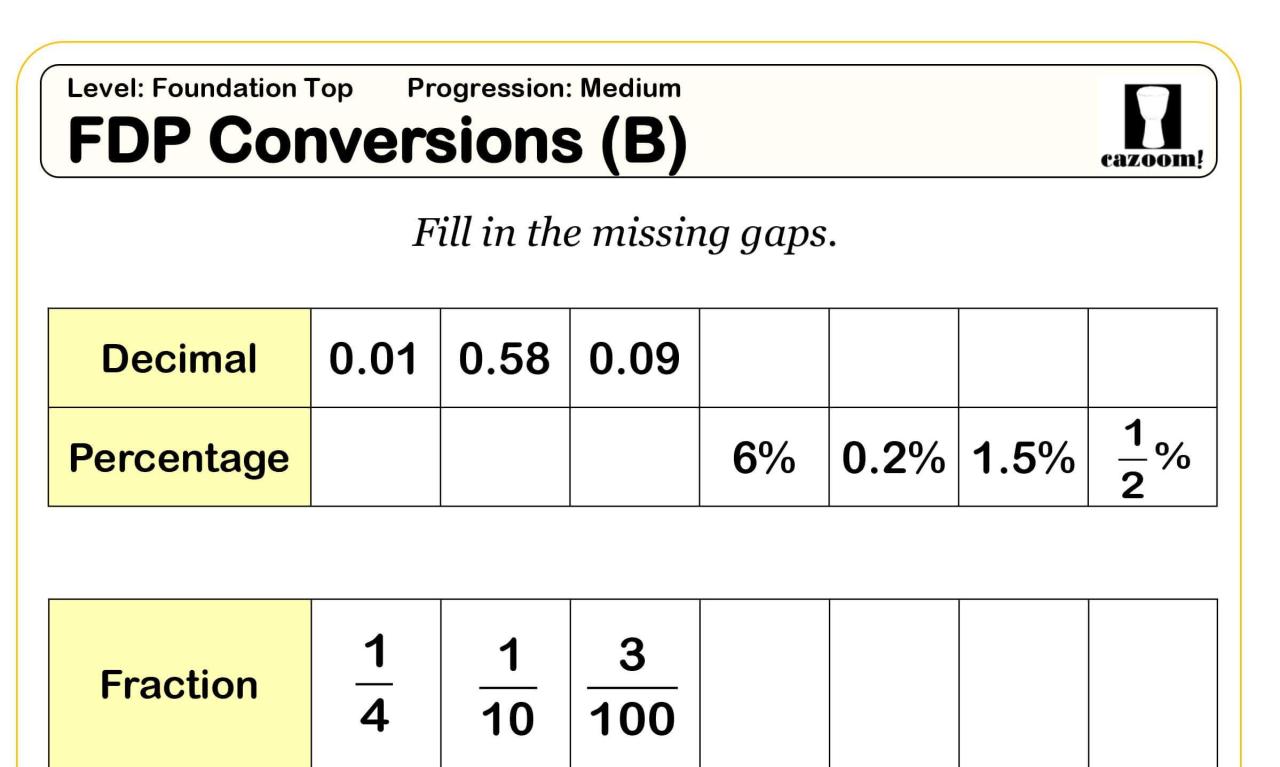
The Algebra 2 12.2 worksheet delves into the intriguing world of rational and irrational numbers, a fundamental concept that plays a pivotal role in mathematical comprehension and beyond.

Grasping these concepts is paramount for academic success, as they form the foundation for more advanced mathematical endeavors. They enhance problem-solving abilities, foster logical reasoning, and pave the way for future explorations in fields such as science, technology, engineering, and finance.

Rational and Irrational Numbers

Rational numbers are numbers that can be expressed as a fraction of two integers, such as 1/2, -3/4, or 0.5. Irrational numbers, on the other hand, are numbers that cannot be expressed as a fraction of two integers, such as √2, π, or e.

These numbers have an infinite, non-repeating decimal expansion.

Understanding the distinction between rational and irrational numbers is crucial for performing operations such as addition, subtraction, multiplication, and division. It also enables students to identify and classify numbers effectively, a skill that proves invaluable in various mathematical contexts.

Operations with Rational and Irrational Numbers

The worksheet delves into the operations of addition, subtraction, multiplication, and division with rational and irrational numbers. It emphasizes the importance of understanding the properties of these operations to ensure accurate calculations and problem-solving.

Students will learn the rules for adding, subtracting, multiplying, and dividing rational and irrational numbers, including the special cases that arise when dealing with mixed operations involving both types of numbers.

: Algebra 2 12.2 Worksheet Answers

Set theory is the branch of mathematics that studies sets, which are well-defined collections of distinct objects. Sets are used to model a wide variety of real-world phenomena, such as the set of all students in a class, the set of all natural numbers, or the set of all prime numbers.

Real-World Applications

Set theory has a wide range of real-world applications, including:

• Computer science:Sets are used to represent data structures such as arrays, lists, and graphs.
• Statistics:Sets are used to represent populations and samples.
• Economics:Sets are used to represent sets of goods and services.
• Biology:Sets are used to represent sets of genes and proteins.

Connections to Other Areas of Mathematics, Algebra 2 12.2 worksheet answers

Set theory is closely connected to other areas of mathematics, including:

• Logic:Sets are used to represent logical propositions.
• Algebra:Sets are used to represent groups, rings, and fields.
• Topology:Sets are used to represent topological spaces.

: Algebra 2 12.2 Worksheet Answers

The following table summarizes the key formulas and theorems related to the concepts in Algebra 2 Worksheet 12.2:

Formulas

Formula	Description
\(y = mx + b\), where \(m\) is the slope and \(b\) is the y-intercept	Equation of a linear function
\(y y_1 = m(x x_1)\), where \(m\) is the slope and \((x_1, y_1)\) is a point on the line	Point-slope form of a linear equation
\(y = \fracy_2 y_1x_2 x_1(x x_1) + y_1\), where \((x_1, y_1)\) and \((x_2, y_2)\) are two points on the line	Two-point form of a linear equation
\(Ax + By = C\), where \(A\), \(B\), and \(C\) are constants	Standard form of a linear equation
\(y = a(x h)^2 + k\), where \(a\), \(h\), and \(k\) are constants	Equation of a parabola
\(y = a|x h| + k\), where \(a\), \(h\), and \(k\) are constants	Equation of an absolute value function
\(y = a\sin(bx + c) + d\), where \(a\), \(b\), \(c\), and \(d\) are constants	Equation of a sine function
\(y = a\cos(bx + c) + d\), where \(a\), \(b\), \(c\), and \(d\) are constants	Equation of a cosine function

Theorems

Theorem	Description
Slope-Intercept Form Theorem	Every non-vertical line has an equation in slope-intercept form.
Point-Slope Form Theorem	The equation of a line passing through a given point with a given slope can be written in point-slope form.
Two-Point Form Theorem	The equation of a line passing through two given points can be written in two-point form.
Standard Form Theorem	Every linear equation can be written in standard form.
Vertex Form Theorem	The equation of a parabola can be written in vertex form.
Absolute Value Function Theorem	The equation of an absolute value function can be written in absolute value form.
Sine Function Theorem	The equation of a sine function can be written in sine form.
Cosine Function Theorem	The equation of a cosine function can be written in cosine form.

: Algebra 2 12.2 Worksheet Answers

Approaching problems related to the concepts in Algebra 2 12.2 worksheet involves a step-by-step process that requires a clear understanding of the concepts and their applications.

First, it’s essential to identify the type of problem you’re dealing with. This will help you determine the appropriate approach and techniques to solve it. For instance, if you’re dealing with a problem involving linear equations, you’ll need to use techniques like substitution or elimination to find the solution.

Once you’ve identified the problem type, the next step is to analyze the given information. This involves carefully examining the problem statement, identifying the variables involved, and understanding the relationships between them.

Based on your analysis, you can start developing a strategy to solve the problem. This may involve setting up equations, applying mathematical operations, or using specific formulas or theorems.

As you work through the problem, it’s important to check your progress and ensure that your steps are leading you towards a solution. If you encounter any difficulties, don’t hesitate to review the concepts or seek assistance from a teacher or tutor.

Finally, once you’ve solved the problem, take a moment to reflect on your approach and identify any areas where you can improve your problem-solving skills. This will help you become more proficient in solving future problems.

Example

Let’s consider a simple problem: Solve for x in the equation 2x + 5 = 13.

To solve this problem, we can follow these steps:

1. Subtract 5 from both sides of the equation: 2x + 5
   • 5 = 13
   • 5
2. Simplify: 2x = 8
3. Divide both sides by 2: 2x/2 = 8/2
4. Simplify: x = 4

Therefore, the solution to the equation 2x + 5 = 13 is x = 4.

Practice Problems for Algebra 2 12.2

The following practice problems are designed to reinforce the concepts covered in the Algebra 2 12.2 worksheet. These problems vary in difficulty to accommodate different learning levels.

Easy Problems

• Simplify the expression: (2x + 3)(x – 5)
• Solve the equation: 2x ²– 5x + 2 = 0
• Factor the expression: x ²– 9

Medium Problems

• Simplify the expression: (x – 2)(x + 2) ²
• Solve the equation: x ³– 8 = 0
• Factor the expression: x ³+ 27

Hard Problems

• Simplify the expression: (x ²– 4)(x ²+ 4)(x ²– 16)
• Solve the equation: x ⁴– 16 = 0
• Factor the expression: x ⁴+ 16

6.

Solving problems related to the worksheet concepts can be approached in various ways, each with its own advantages and disadvantages. Here are a few methods to consider:

1. Algebraic Method

This method involves using algebraic equations to represent the problem and solve for the unknown variables. It is suitable for problems with well-defined mathematical relationships and clear equations.

Advantages:

• Systematic and logical approach
• Provides a step-by-step solution
• Can be used to solve complex problems

Disadvantages:

• Can be time-consuming
• Requires strong algebraic skills
• May not be suitable for all types of problems

2. Graphical Method

This method involves plotting graphs of the relevant functions or equations and analyzing their intersections or other properties. It is particularly useful for problems involving optimization or finding solutions to systems of equations.

Advantages:

• Provides a visual representation of the problem
• Can quickly identify solutions or trends
• Suitable for problems with non-linear functions

Disadvantages:

• May not be precise enough for some applications
• Requires graphing skills and tools
• Can be difficult to interpret graphs accurately

3. Numerical Method

This method involves using numerical approximations to find solutions. It is often used when analytical methods are not feasible or when dealing with large datasets.

Advantages:

• Can provide approximate solutions quickly
• Suitable for problems with complex functions
• Can be implemented using computers or calculators

Disadvantages:

• May not be as accurate as analytical methods
• Can be computationally expensive for large problems
• Requires understanding of numerical algorithms

4. Trial and Error Method

This method involves making educated guesses and checking if they satisfy the problem’s conditions. It is often used as a starting point for more refined methods or when other methods are not applicable.

Advantages:

• Simple and easy to implement
• Can provide initial approximations
• Suitable for problems with limited information

Disadvantages:

• Can be inefficient and time-consuming
• May not lead to optimal solutions
• Not suitable for complex problems

: Algebra 2 12.2 Worksheet Answers

Common misconceptions and errors when working with the concept of in Algebra 2 include:

* -*Misconception 1: Students may assume that can be used to represent any set of numbers. However, specifically represents the empty set, which contains no elements.

Strategy: Emphasize that is a unique symbol that represents the empty set and cannot be used to represent any other set.

* -*Misconception 2: Students may struggle to understand the concept of the empty set. They may believe that the empty set is the same as the set 0 or the set .

Strategy: Explain that the empty set is a special set that contains no elements. It is different from the set 0, which contains the single element 0, and the set , which is also empty but is not the same as .

* -*Misconception 3: Students may have difficulty applying the properties of the empty set. They may not realize that the empty set is a subset of every other set and that the union of the empty set with any other set is that set.

Strategy: Provide examples to illustrate the properties of the empty set. Show students that the empty set is a subset of the set 1, 2, 3 because it contains no elements that are not in 1, 2, 3. Also, show that the union of the empty set with the set 1, 2, 3 is 1, 2, 3 because the empty set does not add any elements to 1, 2, 3.

Quick FAQs

What are the key formulas covered in Algebra 2 12.2?

The worksheet covers essential formulas such as the quadratic formula, vertex formula, and factoring formulas.

How can I apply these concepts to real-world situations?

Algebraic concepts find applications in fields like engineering, finance, and physics, enabling us to model and solve problems in diverse contexts.